Geometry In Space

What is the shape of the Universe? An interesting question, for sure, and one that carries with it other implications - like what the ultimate fate of the Universe will be. For many years, scientists have proposed that there are three possibilities for the curvature (or shape) of the Universe. The Universe can be flat, like a piece of paper. Or it can have a positive curvature, like a sphere. Or it can have a negative curvature, like the middle part of a horse's saddle. To a certain degree, the curvature the Universe has depends on how much mass there is in the Universe...and the amount of mass will determine its ultimate fate. So, it is of more than just a passing fancy that we might want to measure the curvature of the Universe. But how exactly do you do that?

In this lesson, we will explore one possible way to measure the curvature of the Universe, namely, by measuring the sum of the angles in a triangle in the Universe. Depending on the shape of the Universe, the sum will be either less than, equal to, or greater than 180 degrees. Of course, we can't measure just ANY triangle...we have to use a very big triangle in order to be able to discern the shape of the Universe. Every triangle will give the result of 180 degrees if you measure just a teeny tiny part of a very big space...no matter what its curvature is.

Flat Universe	Positive Curvature Universe	Negative Curvature Universe

So how do you measure a very big triangle in the Universe? That will be discussed as well, since it is something that NASA's Microwave Anisotrophy Probe (MAP) mission will do in the next few years. But first, let's learn about different shapes, or geometries, and how they effect the sums of the angles of a triangle. This standards-based topic, called Non-Euclidean Geometry, will challenge your brain - but only because you are used to the flat space and rules of Euclidean Geometry. So be prepared for a mind-bending exercise!

Student Worksheet

• Activity Worksheet

Teacher Notes

When most students study geometry, they learn Euclidean Geometry - which is essentially the geometry of a flat space. Euclidean Geometry is based upon a set of postulates, or self-evident proofs. Such proofs present "on obvious truth that cannot be derived from other postulates." Here are Euclid's postulates:

1. You can draw a straight line between any 2 points.
2. You can extend any segment indefinitely.
3. You can draw a circle with any given point as the center and any given radius.
4. All right angles are equal.
5. That, if a straight line falling on two straight lines makes the interior angles on the same side less than two right angles, the two straight lines, if produced indefinitely, meet on that side on which are the angles less than the two right angles.

The fifth postulate is known as the Parallel Postulate. This Postulate was rewritten by Scottish scientist and mathematician John Playfair (1748-1819) as the following (referred to as the Playfair axiom): "Through a given point not on a given line, there passes at most one line that is parallel to the given line."

It was the obvious difference between the first 4 postulates and the fifth which led mathematicians to believe that in fact the fifth was not a postulate, but could be deduced from the first 4. In trying to do so, they discovered elliptic geometry and hyperbolic geometry!

If you assume the fifth postulate is false, there are 2 possible negations:

1. Through a given line not on a given point, there passes more than one line parallel to the given line.
2. Through a given point not on a given line, there passes no lines parallel to a given line.

The first assumption was investigated by German mathematician Carl Friedrich Gauss (1777-1855), Russian mathematician Nikolai Lobachevsky (1792-1856), and Hungarian mathematician Janos Bolyai (1802-1860). They discovered that it led to a new geometry that did not contradict any of the other postulates. If they replaced the Parallel Postulate with this new assumption, they had discovered the non-Euclidean geometry called hyperbolic geometry.

German mathematician Georg Friedrich Bernhard Riemann (1826-1866) investigated the second assumption. If the Parallel Postulate is replaced with this postulate, again none of the other postulates were violated. The non-Euclidean Geometry this set of postulates creates is called elliptic geometry.

All of the theorems in Euclidean Geometry which do not rely on the Parallel Postulate apply intact in the non-Euclidean geometries. One theorem, perhaps the most famous example, which does rely on the Parallel Postulate is the Triangle Sum Theorem, which states: the sum of the three angles in a triangle is 180°. Since students are probably already familiar with the flat space of Euclidean Geometry and how the angles of a triangle in that space add up to be 180°, we will focus on seeing what triangles look like and behave like in elliptic and hyperbolic spaces.

Elliptic Geometry

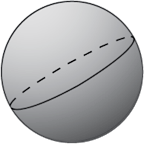You can use the surface of a sphere as a model for elliptic geometry. But if you do, you must change the wording of the Euclidean Postulates slightly. In elliptic geometry, the word "line" is replaced by "great circle". A great circle is defined as any circle on the surface of a sphere for which the diameter passes through the center of the sphere. You also need to define "pole points" to be points on the opposite ends of any diameter of the sphere. To create elliptic geometry, Riemann assumed that through a given point not on a line, there passes no lines parallel to that given line. This new geometry introduces a few changes. In Euclidean geometry, lines are infinite in length. In elliptic geometry, great circles never end, but they are finite in length. A line segment is now defined as an arc of a great circle, or the distance along the great circle between two points. When a straight line is extended, its ends eventually meet forming a great circle. Any two "straight lines" eventually meet, since any two great circles intersect at pole points. One result of this change in the Parallel Postulate is that the Triangle Sum Theorem has a different ending: The sum of the three angles in a triangle in elliptic geometry is always greater than 180°.

To find a model for a hyperbolic geometry, we need one in which for every line and a point not on that line, there is more than one parallel line. French mathematician Henri Poincaré (1854-1912) came up with such a model, called the Poincaré disk. The Poincaré disk consists of all the points on the interior of a circle. Lines in this geometry are arcs inside the circle, but not just any arcs. The arcs must have their ends on the circumference of the circle and must be perpendicular to the circle at both end points. As you may have guessed, this geometry also changes the ending of the Triangle Sum Theorem so that it reads: The sum of the three angles of a triangle in this geometry is always less than 180°.

Let's Investigate Non-Euclidean Geometries!

• Student Worksheet
• Models for Euclidean, Elliptic, and Hyperbolic Geometries. For example, pieces of paper, large styrofoam spheres (found in craft stores) or globes, and Poincare disks or hyperbolic sheets (see Appendix A)
• String cut into several different lengths, a few inches, a foot, three feet, etc. Tie each piece of string into a loop.
• Protractors
• Tape or tacks to hold string in place on models
• Paper and pencil to record measurements

1. Have students form small groups.
2. Give each group a Student worksheet and one of the lengths of string, spreading the different lengths around the room. Have a different length of string for each group.
3. Have the students first make a triangle on a flat piece of paper using the string to form the perimeter. Use tacks or tape to secure the vertices.
4. Students should measure and add the angles of their triangle. They should see that their angles add up to 180 degrees.
5. Next, have students make new triangles on the paper until they are convinced that no matter what shape triangle they form, the total angle summation will equal 180 degrees.
6. Now have them make triangles on the Elliptic Model, using the string as the perimeter.
7. Have them stretch the string as tightly on the elliptic model as they can. Remember, the elliptical model you will use is a sphere.
8. Students may find that the sides of their triangle are not straight lines. This is because on the surface of a sphere the shortest distance along the surface is not a straight line. It is actually a portion of what is called a Great Circle. Remember, a Great Circle is a circle on the surface of a sphere that has the center of the sphere as its center. Remind students that, on a globe, all of the lines of longitude are Great Circles, but the only line of latitude (which is also a Great Circle) is the Equator.
9. Have students measure the angles of their triangle and record the sum of the angles. Repeat this step several times for different triangles. Students should find that the sum of the angles of each triangle add up to more than 180 degrees.
10. With your hyperbolic model (either the Poincaré disk or the hypersheet), try the same procedure. Remember that your lines are special arcs in this geometry. To measure the angle formed by two lines, measure the angle formed by the tangents to the arcs at the intersection points. Students should find that the sum of the angles of each triangle should add up to less than 180 degrees.
11. Have the students tabulate their results on a chalkboard in the front of the room for each of the surfaces. See if they can find trends based upon the length of the string and the sum of the angles. In Euclidean geometry, it should not matter how long the string is, the angles should always add up to 180 degrees. In hyperbolic geometry, the shorter the string (the smaller the perimeter of the triangle) the closer the sum of the angles is to 180 degrees, but the sum should always remain less than 180 degrees. For elliptic geometry, the shorter the perimeter of the triangle the closer the sum of the angles should be to 180 degrees, but the sum should always be greater than 180.
12. Students should discuss how each of these geometries could change our view of the Universe. Remind them that not only could it change our perspective, but it will also tell the ultimate fate of our Universe. Kind of like a geometric fortune teller!

Additional Resources

• For a more complete discussion of Euclidean and Non-Euclidean Geometries and reasons to teach Non-Euclidean Geometries in your classroom please visit http://math.rice.edu/~joel/NonEuclid/NonEuclid.html.This website also contains a web-based program, which your students can use to investigate a Poincaré Disk.

National Standards

Science

• Origin and Evolution of the Universe: In grades 9 -12 all students should understand that:

  "Early in the history of the universe, matter, primarily in the form of hydrogen and helium, clumped together by gravitational attraction to form countless trillions of stars. Billions of galaxies, each of which is a gravitationally bound cluster of billions of stars, now form most of the visible mass of the universe; Stars produce energy from nuclear reactions, primarily the fusion of hydrogen to form helium. These and other processes in stars have led to the formation of all the other elements."

• Nature of Scientific Knowledge

Mathematics

• Analyze properties and determine attributes of two-and three-dimensional objects;
• Establish the validity of geometric conjectures using deduction, prove theorems, and critique arguments made by others;
• Use geometric models to gain insights into, and answer questions in, other areas of mathematics;
• Use geometric ideas to solve problems in, and gain insights into, other disciplines and other areas of interest such as art and architecture.
• Develop an understanding of an axiomatic system through investigating and comparing Euclidean and Non-Euclidean geometries